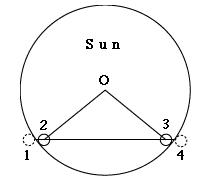
Predictions for the transit give four "contact" times, at which the dark Venus disk touches the edge of the Sun's disk (marked by numbers on Figure 3)"

   
First contact - Venus disk touches the Sun from the outside, on entry
Second contact - Venus disk touches the Sun from the inside, on entry
Third contact - Venus disk touches the Sun from the inside, on exit
Fourth contact - Venus disk touches the Sun from the outside, on exit

Fig. 3   The 4 contacts of Venus and the Sun's disk

    Obviously, Venus is not visible during 1st and 4th contacts, so we use 2nd contact to define A or A' and 3rd contact to define B or B' (see Figure).

    The Sun's disk has an apparent diameter 2R of about 31.5 minutes of arc, and the radius r of Venus is about 1 minute. As noted for AB etc. on a preceding page, R and r are not distances but visual angles. Still, in this calculation all angles can be handled like distances, because all are very small. You may imagine Figure 3 projected on the inside of a huge sphere (e.g. planetarium dome, or the celestial sphere itself). An area of half a degree, which is the approximate diameter of the Sun, covers such a small part of the entire sphere that to a good approximation it can be viewed as flat, and distances on it are very nearly proportional to the angles which they cover.

    We choose the points A and B to be not on the edge ("limb") of the Sun's disk during 2nd and 3rd contact, but at the center of Venus at those times. If O is the center of the Sun, these points lie on a circle around O with radius (R–r). Thus the time for covering AB marks the passage of Venus across a chord (=straight cut across a circle) of a "reduced Sun" covering only 30.5 minutes of arc.

    We will also need a moderately accurate value of the distance h of the line AB from the center O, or more conveniently the angle θ (Greek theta; see Fig. 4 below), half the angle covered by AB. Deriving it from published photographs (e.g. the published version of Fig. 1 of the preceding page, or plots like the one provided by the U. of Lancashire) gives about 46.4 degrees. Then (Figure 4 below)

Fig. 4   Variables used in the calculation

Since the transit time T is proportional to the distance (AB), we can write

where k is some proportionality factor. Its value will not be needed, since we aim to use the ratio between T and the difference ΔT in transit times observed at two locations, and in deriving the ratio, k cancels out. Using trigonometry

    If the line A'B' of the other station is slightly longer, it covers a slightly larger angle 2(θ+δ), and its distance from O is not h but

    If δ is a very small angle, sinδ is also small. But even when a small value of sinδ remains important enough to be included, cosδ is probably so close to 1 that one can safely replace it by 1. To demonstrate this, note that

    Recalling that the square root is also the (1/2) power, by the binomial theorem

    So if we approximate cosδ by 1, the error made is only of the order of sin²δ which (as is true for numbers much smaller than 1) is much smaller than sinδ . If in expressing h', we keep sinδ but replace cosδ with 1, then to a good approximation

    The transit time at point P' is obtained in a similar way to equation (2)

    and to the same good approximation as before, with the angle δ small

    This is just about what was expected: the smallness of the ratio on the left is balanced by the smallness of sinδ on the right. Extracting that term

    This can be substituted in equation (4) for D, another small term

    That is what was needed. As Halley suggested, the separation D between transit tracks is now expressed in terms of the observed difference between transit times, as well as the length of the observed transit time T (or its average (T + T')/2) as well as the angle θ, giving the distance of the transit path from the center of the Sun